In this article, we shall discuss the response of a purely resistive circuit to alternating current supply, i.e. AC through a pure resistance.
Consider an alternating voltage source v is applied across a resistor of resistance R as shown in figure-1.
Let the alternating voltage is given by the following expression,
According to Ohm’s law, the electric
current flowing through the resistor is given by,
`\i=v/R`
`\⇒i=(V_m sinωt)/R`
`\∴i=I_m sinωt…(2)`
Where Im = Vm/R is
the maximum value of the current through the resistor R.
From equations (1) and (2), it is clear
that in pure resistance, the voltage and current are in the same phase. The
phasor diagram for the purely resistive AC circuit is shown in figure-2.
The phasor diagram shows that the phase angle between the voltage across and current through a resistance is zero.
Power Relation for Purely Resistive AC Circuit:
The instantaneous power consumed by the
resistance is given by,
`\p=v.i`
`\⇒p=(V_m sinωt )(I_m sinωt )`
`\⇒p=V_m I_m sin^2ωt`
`\∵sin^2θ=(1-cos2θ)/2`
`\∴p=(V_m I_m)/2 (1-cos2ωt )…(3)`
Therefore, the total average power
consumed by the resistor in one cycle is given by,
`\P=1/(2Ï€) ∫_0^(2Ï€) (p.dωt)`
`\⇒P=1/(2Ï€) ∫_0^(2Ï€) (V_m I_m)/2 (1-cos2ωt ).dωt`
`\⇒P=(V_m I_m)/(4Ï€) ∫_0^(2Ï€) 1.dωt-(V_m I_m)/(4Ï€) ∫_0^(2Ï€) cos(2ωt).dωt`
`\⇒P=(V_m I_m)/(4Ï€) [(1)-(sin2ωt )]_0^(2Ï€)`
`\⇒P=(V_m I_m)/(4Ï€) (2Ï€)`
`\∴P=(V_m I_m)/2=V_m/\sqrt{2} I_m/\sqrt{2}`
`\⇒P=VI…(4)`
Where V and I be the RMS value of
applied voltage and resulting current respectively. From equation (3), it can be
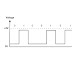
noted that the instantaneous power cannot be negative, and it has two components,
i.e. a constant component and a pulsating component having a frequency double the supply voltage.
The double frequency alternating component of the instantaneous power is equal to zero at ωt = 0° and 180°, and it is maximum at ωt = 90° and 270° as shown in figure-3.
Hence, this is all about alternating
current through pure resistance.
.png)
