What is the Basic Terminology of AC Circuit?

In this article, we will discuss the basic terms related to AC (Alternating Current) circuits.

Knowledge of this terminology is very important to study AC circuits. Hence, every electrical engineering student and professional must know the meaning of these basic terms of AC circuits.

In the upcoming sections of this article, we will discuss the following six major terms related to ac circuits:

• Waveform
• Cycle
• Time Period
• Frequency
• Phase Angle
• Phase Difference

Now, let us discuss these terms of AC circuits in detail one by one.

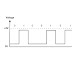
(1). Waveform:

The curve or graph obtained by plotting instantaneous values of an AC quantity (current or voltage) against time is called the waveform of the AC quantity.

Consider a sinusoidally varying alternating voltage expressed by,

`\v=V_m  sin⁡ωt`

The waveform of this alternating voltage is shown in figure-1 below.

(2). Cycle:

One complete set of positive and negative instantaneous values of an alternating wave is called a cycle. In other words, one complete set of alterations is called the cycle of the ac wave. One complete cycle of the alternating voltage (v) is shown in figure-1 above.

Here, in one complete cycle, there are two half cycles, i.e. one positive half cycle and one negative half cycle.

(3). Time Period:

The time taken to complete one cycle by the AC wave is called its time period. The time period is usually denoted by T.

For the AC wave shown in figure-1, the time period is equal to 2π. In other words, for a sinusoidal ac wave, during one time period T, the change in the angle of the wave is equal to 2π. Therefore,

`\θ=ωT=2π`

`\∴T=(2π)/ω`

The time period is measured in seconds (s).

(4). Frequency:

The number of cycles completed by the AC wave in one second is called the frequency of the AC wave. Frequency is denoted by “f”.

The ac wave shown in figure-1, one cycle is completed in T seconds. Therefore, in 1-second 1/T cycles will be completed. Hence, the frequency is given by,

`\f=1/T`

Frequency is measured in cycles per second (c/s). The SI unit of frequency is Hertz (Hz).

Since, we have,

`\T=(2π)/ω`

Therefore, the frequency can also be given by,

`\f=ω/(2π)`

Thus, the relationship between angular frequency (ω) and linear frequency (f) is given by,

`\ω=2πf`

Where the angular frequency ω is measured in radians per second.

(5). Phase Angle:

The angle of an AC wave at t = 0 is called the phase angle of the AC wave. For example, consider an ac wave expressed by the following expression,

`\i=I_m  sin⁡(ωt+ϕ)`

At any instant of time (t), the angle of the wave is equal to (ωt + ϕ). But at t = 0, we have, the angle of the wave equal to ϕ. Therefore, ϕ is called its phase angle.

(6). Phase Difference:

The angular separation between the zero crossing points of two ac waves is called the phase difference between them.

Let us consider three sinusoidal current waves of the same magnitude,

`\i_1=I_m  sin⁡ωt`

`\i_2=I_m  sin⁡(ωt+θ_1)`

`\i_3=I_m  sin⁡(ωt-θ_2)`

The waveform representation of these currents is shown in the figure-2 below.

Here, it can be seen that the current i₁ starts at t = 0, thus, it may be taken as the reference wave.

By definition, the angular distance between zero crossing points of two waves is called phase difference. Thus, the angle θ₁ is the phase difference between i₁ and i₂, and θ₂ is the phase difference between i₁ and i₃.

The phase difference between two ac waves can be numerically computed by finding the difference in the phase angles of the two waves.

For example, from the figure-2, we have,

Phase difference between i₂ and i₁ = Δθ = θ₁ – 0

`\⇒Δθ=θ_1`

In general,

`\Δθ=θ`

• If Δθ is positive, then i₂ is said to be leading the current wave i₁ by an angle of Δθ.
• If Δθ is negative, then i₂ is said to be lagging the current wave i₁ by an angle of Δθ.

Hence, in figure-2, we can conclude,

• The wave i₂ leads i₁ by an angle θ₁.
• The wave i₃ lags i₁ by an angle θ₂.
• The wave i₂ leads i₃ by an angle (θ₁ + θ₂).
• The wave i₃ lags i₂ by an angle (θ₁ + θ₂).

In general, if the phase angle of an AC wave is θ, it is said to be leading from the reference wave. On the other hand, if the phase angle of an AC wave is –θ, it is said to be lagging from the reference wave.

Therefore, this is all about the six most important terms associated with AC circuits in electrical engineering.